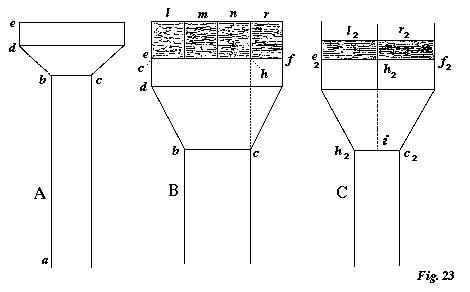
142 THE STONES OF VENICE CONSTRUCTION

(1.) The height of the shaft, a b;

(2.) Its diameter, b c;

(3.) The length of slope of bell, b d;

(4.) The inclination of this slope, or angle c b d;

(5.) The depth of abacus, d e.

For every change in any one of these quantities we have a new proportion of capital: five infinites, supposing change only in one quantity at a time; infinity of infinities in the sum of possible changes.

It is, therefore, only possible to note the general laws of

change; every scale of pillar, and every weight laid upon it, admitting, within certain limits, a variety, out of which the architect has his choice; but yet fixing limits which the proportion becomes ugly when it approaches, and dangerous when it exceeds. But the inquiry into this subject is too difficult for the general reader, and I shall content myself with proving four laws, easily understood and generally applicable; for proof of which if the said reader care not, he may miss the next four paragraphs without harm.

§ 14. (1.) The more slender the shaft, the greater, proportionally, may be the projection of the abacus. For, looking back to Fig. 23, let the height a b be fixed, the length d b,

[Version 0.04: March 2008]